VIRTUAL MAPS OF
 MANDELBROT AND
MANDELBROT AND
 JULIA SETS
JULIA SETS
(1996)
The following pages
represent a new section of my Fractal Gallery.
Here I want to present an alternative graphical way to navigate across
Mandelbrot and Julia sets, based on the technique of CLICKING virtual
maps.
Together with each map and image you will find some explanation related
to the parameters and computer programs.
 Choose the map you are interested in! Or, if you like, see all of them...
Choose the map you are interested in! Or, if you like, see all of them...
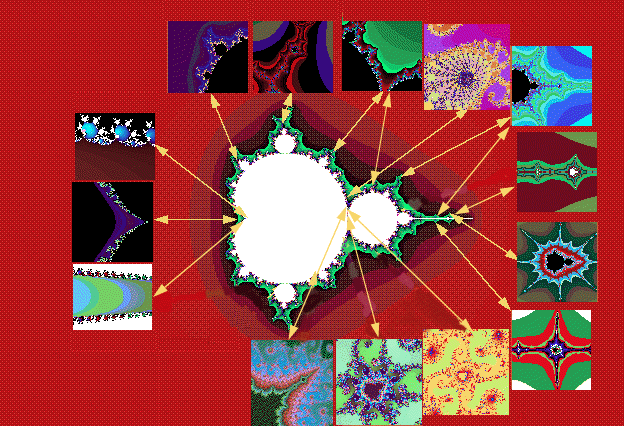
 To navigate into the Mandelbrot set click the Mandelbrot
zoom map
To navigate into the Mandelbrot set click the Mandelbrot
zoom map
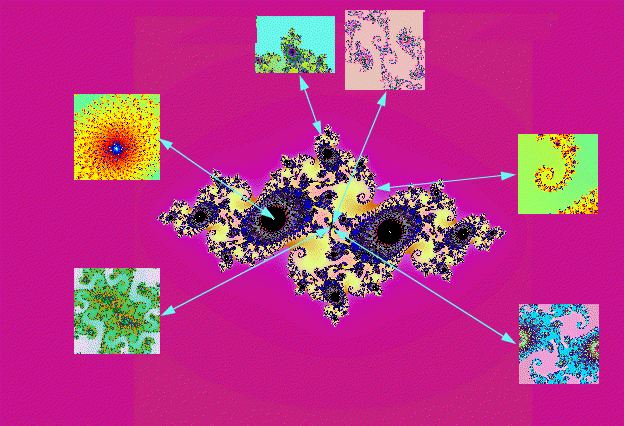
 To navigate into into the Seahorse Julia set click the Julia zoom map
To navigate into into the Seahorse Julia set click the Julia zoom map
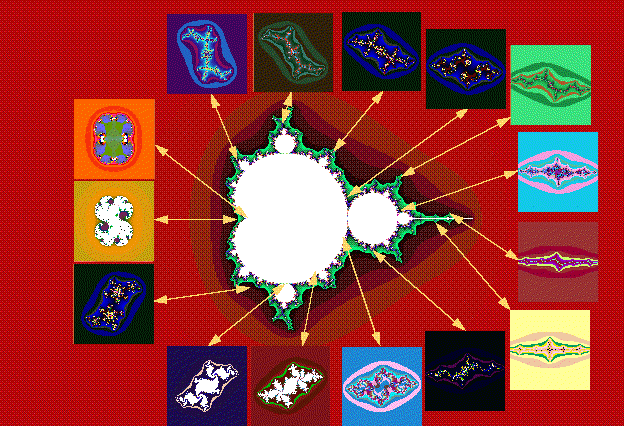
 For a tour of Julia sets along the boundary of Mandelbrot set
For a tour of Julia sets along the boundary of Mandelbrot set
click the Map of Julia sets on Mandelbrot boundary
Click
on one of the small images into the Map to see the magnification of the Mandelbrot
set in full size. You will notice that the colour palette has
been chosen differently for each image to reach the best chromatic effect.
In this
map I propose some magnified images of the Seahorse Julia set, the
most spectacular of the Julia sets, which is obtained when the complex constant
parameter c = 0.74543 + i 0.11301.
The
Mandelbrot set is a sort of book each page of which is a picture
of a Julia set, corresponding to a value of the parameter c identifying
a point of the Mandelbrot set.
In a
more mathematical language we say that each Julia set may be represented by
a point in a complex parameter space: each point of this space lies inside
the Mandelbrot set if the Julia set is connected, while it lies outside
the Mandelbrot set when the corresponding Julia set is not connected
(Fatou dust). When the point labelled by the parameter c runs
along the fractal boundary of the Mandelbrot set, the Julia set modifies its
shape in a very elegant way.
The
map I present here shows some of the shapes of the Julia sets corresponding
to values of c moving in a neighbourhood of the boundary of the Mandelbrot
set.
BACK
to FRACTAL GALLERY
BACK TO CIRAM HOME PAGE
 MANDELBROT AND
MANDELBROT AND
![]() JULIA SETS
JULIA SETS![]() JULIA SETS
JULIA SETS![]() Choose the map you are interested in! Or, if you like, see all of them...
Choose the map you are interested in! Or, if you like, see all of them...![]()
![]() To navigate into the Mandelbrot set click the Mandelbrot
zoom map
To navigate into the Mandelbrot set click the Mandelbrot
zoom map ![]() To navigate into into the Seahorse Julia set click the Julia zoom map
To navigate into into the Seahorse Julia set click the Julia zoom map ![]() For a tour of Julia sets along the boundary of Mandelbrot set
For a tour of Julia sets along the boundary of Mandelbrot set

